Moved here from lemmy.world. Long live piracy!
- 3 Posts
- 48 Comments

 8·2 months ago
8·2 months agoIn criminal cases. In civil suits someone always loses, so an average person cannot win more than 50% of cases.

 7·2 months ago
7·2 months agoFinally the mathematically correct answer! Thank you for typing this out
Afaik dreams get erased because you move and your brain tries to switch to day mode (so that your dream experiences don’t confuse you when you’re awake). The easiest way to write down your dreams precisely is to try and wake up while still laying in bed, and write down your dreams when you’re not as eepy but still in bed.

 10·5 months ago
10·5 months agoMads Mikkelsen
Also called “real estate”
If the licence is already open source then they can’t do shit. Unfortunately, they have other methods of discouraging programmers from working on the project, but ultimately open source will prevail.
You accidentally added a dot at the end of the link. Here’s the fixed one https://google.github.io/styleguide/cppguide.html

 7·7 months ago
7·7 months agoDNS over HTTPS and use a DNS located in another country - problem solved
Almost correct - that would be acceleration. Jerk is the third derivative of position with regard to time, so if the number is speed, the jerk is the rate of the rate of the number go up go up go up
The main verb is most often in the second position, the second verb you are referring to is a placeholder for an auxiliary verb that usually is a different form of a previously main verb
Remember kids, piracy is not only moral, but a moral obligation in this capitalist hellscape! (and not theft by definition, and should not be illegal) Torrents are one of the few effective weapons against corporations
I think if it was democratic, I would trust it even less. Relying on democracy in a community when specialist knowledge is required to understand a topic is a major factor that contributed to the creation of such abominations as anti-vaxxers, flat-earthers etc.

 2·10 months ago
2·10 months agoWell, sets of measure 0 are one of the fundamentals of the whole integration theory, so it is always wise to pay particular attention to their behaviour under certain transformations. The whole 1 + int + int^2 + … series intuitively really seems to work as an inverse of 1 - int over a special subspace of R^R functions, I think a good choice would be a space of polynomials over e^x and X (to leave no ambiguity: R[X, e^X]). It is all we need to prove this theorem, and these operators behave much more predictably in it. It would be nice to find a formal definition for the convergence of the series, but I can’t think of any metric that would scratch that itch.

 3·10 months ago
3·10 months agoInt is definitely not injective when you consider noncontinuous functions (such as f(X)={1 iff X=0, else 0}). If you consider only continuous functions, then unfortunately 1-Int is also not injective. Consider for example e^x and 2e^x. Unfortunately your idea with equivalence classes also fails, as for L = 1 - Int, L(f) = L(g) implies only that L(f-g) = 0, so for f(X)=X and g(X)=X + e^x L(f) = L(g)

 3·10 months ago
3·10 months agoAfter careful consideration I have come to the conclusion that the inverse of the operator L is obviously not 1/L and you are absolutely right. This derivation is complete nonsense, my apologies. In fact no such inverse can even exist for the operator 1 - integral, as this function is not an injection.

 17·10 months ago
17·10 months agoExcept the first assumption that e^x = its own integral, everything else actually makes sense (except the DX are in the wrong powers). You simply treat the “1” and “integral dx” as operators, formally functions from R^R into R^R and “(0)” as calculating the value of the operator on a constant-valued function 0. EDIT: the step 1/(1-integral) = the limit of a certain series is slightly dubious, but I believe it can be formally proven as well. EDIT 2: I was proven wrong, read the comments

 26·1 year ago
26·1 year agoIt might be illegal in your country, but you can consider generating a person’s face (and details) and creating a fake ID photo using it to protect your identity.

 2·1 year ago
2·1 year agoIt would appear this is the simplest solution: https://www.microsoft.com/en-us/download/details.aspx?id=102134
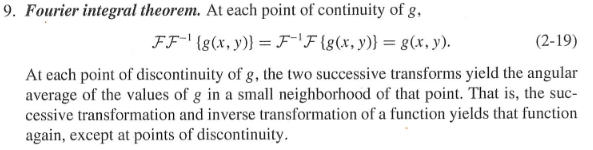







Because that’s the point